What Is A Mixed Number? A Comprehensive Guide You Won’t Want To Miss

Luis McKenzie III 11 May 2025

Alright folks, let’s dive right into something that’s both fascinating and practical for anyone brushing up on their math skills. What is a mixed number? If you’re scratching your head or haven’t thought about this since middle school, you’re not alone. But don’t worry, because we’re about to break it down in a way that’ll make you feel like a math wizard. So grab your favorite snack, get comfy, and let’s demystify mixed numbers together.

Now, why should you care about mixed numbers? Well, they’re everywhere! From baking recipes to splitting bills with friends, understanding mixed numbers can save you from math-induced headaches. Whether you’re a student, a parent helping with homework, or just someone curious about how numbers work, this guide will give you all the tools you need. Trust me, by the end of this, you’ll be solving problems faster than you can say “pi.”

So buckle up, because we’re diving deep into the world of fractions, whole numbers, and everything in between. Let’s make math fun again, shall we?

Defining a Mixed Number
A Brief History of Mixed Numbers
Converting Mixed Numbers
Adding Mixed Numbers
Subtracting Mixed Numbers
Multiplying Mixed Numbers
Dividing Mixed Numbers
Real-Life Examples of Mixed Numbers
Tips and Tricks for Working with Mixed Numbers
Common Mistakes to Avoid

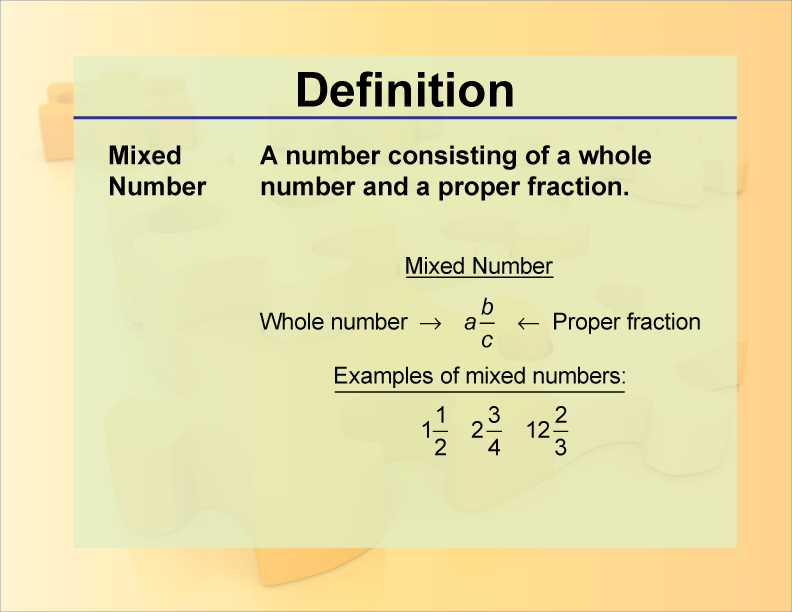
Defining a Mixed Number

A mixed number, in its simplest form, is a combination of a whole number and a fraction. Think of it like having a pizza where you’ve eaten two whole pies and only half of another one. Instead of saying “two and a half pizzas,” we write it as 2 1/2. Simple, right? But there’s more to it than just writing numbers together.

Mixed numbers are super useful when dealing with quantities that aren’t whole. For example, if you’re measuring ingredients for a recipe or splitting a bill, mixed numbers make it easier to understand exactly what you’re working with. So, whether you’re a math whiz or just starting out, mastering mixed numbers is a skill that’ll stick with you for life.

How Are Mixed Numbers Used in Daily Life?

Let’s be real—math isn’t just for textbooks. Mixed numbers pop up in all kinds of everyday situations. Imagine cutting a loaf of bread into slices and realizing you’ve eaten three slices plus half of another one. You’d say you ate 3 1/2 slices, and boom—you’ve just used a mixed number! It’s all about making sense of parts and wholes in a way that’s easy to grasp.

A Brief History of Mixed Numbers

Believe it or not, mixed numbers have been around for centuries. Ancient civilizations like the Egyptians and Babylonians used fractions to solve practical problems, like dividing land or sharing resources. Over time, mathematicians refined these concepts, and mixed numbers became a staple in arithmetic.

Why does history matter? Well, understanding where mixed numbers come from gives us a deeper appreciation for how far math has come. Plus, it’s kinda cool to know that the same principles used by ancient mathematicians are still relevant today. So next time you’re working with mixed numbers, remember—you’re part of a long tradition of problem solvers!

Evolution of Fractional Notation

Fractions, including mixed numbers, haven’t always looked the way they do now. In fact, early civilizations used different systems to represent parts of a whole. The Egyptians, for instance, preferred unit fractions (fractions with a numerator of 1). It wasn’t until much later that the modern notation we use today was developed. Fun fact: the word “fraction” comes from the Latin word fractus, meaning “broken,” which perfectly describes what fractions represent.

Converting Mixed Numbers

Converting mixed numbers is one of those skills that seems tricky at first but becomes second nature with practice. Let’s say you have the mixed number 3 1/4. To convert it to an improper fraction, follow these steps:

Multiply the whole number (3) by the denominator (4): 3 × 4 = 12.
Add the numerator (1) to the result: 12 + 1 = 13.
Write the new numerator (13) over the original denominator (4): 13/4.

Voila! You’ve converted a mixed number into an improper fraction. This process is super handy when you’re solving equations or simplifying expressions.

Why Convert Mixed Numbers?

Converting mixed numbers isn’t just about following rules—it’s about making math easier. Improper fractions are often simpler to work with in calculations, especially when adding, subtracting, multiplying, or dividing. Plus, converting back and forth between mixed numbers and improper fractions helps reinforce your understanding of how they relate to each other.

Adding Mixed Numbers

Adding mixed numbers might sound intimidating, but it’s actually pretty straightforward once you get the hang of it. Here’s how you do it:

Separate the whole numbers and fractions.
Add the whole numbers together.
Add the fractions together (make sure they have the same denominator).
Combine the results.

For example, if you’re adding 2 1/3 and 1 2/3, you’d first add the whole numbers (2 + 1 = 3) and then the fractions (1/3 + 2/3 = 3/3 = 1). Finally, combine them to get 3 + 1 = 4. Easy peasy!

Tips for Adding Mixed Numbers

Here are a few tips to make adding mixed numbers even smoother:

Always check if the fractions have the same denominator before adding.
If they don’t, find the least common denominator (LCD) and rewrite the fractions accordingly.
Don’t forget to simplify your final answer if possible!

Subtracting Mixed Numbers

Subtracting mixed numbers follows a similar process to adding them, but there’s one extra step to watch out for. Let’s say you’re subtracting 3 1/4 from 5 3/4. First, subtract the whole numbers (5 - 3 = 2). Then, subtract the fractions (3/4 - 1/4 = 2/4 = 1/2). Combine the results to get 2 1/2.

But what if the fraction you’re subtracting is larger than the one you’re subtracting from? In that case, you’ll need to borrow from the whole number. For example, if you’re subtracting 1 3/4 from 2 1/4, you’d borrow 1 from the whole number, making it 1 5/4. Now you can subtract normally and get 1 2/4 = 1 1/2.

Common Challenges in Subtraction

Borrowing can trip people up, but with practice, it becomes second nature. Just remember: always simplify your fractions and double-check your work. Math is all about precision, so take your time and don’t rush!

Multiplying Mixed Numbers

Multiplying mixed numbers is where things get really interesting. To multiply 2 1/2 and 3 1/3, start by converting both to improper fractions (5/2 and 10/3). Then, multiply the numerators (5 × 10 = 50) and the denominators (2 × 3 = 6). Your result is 50/6, which simplifies to 8 2/6 = 8 1/3.

See how that works? Multiplying mixed numbers is all about converting, multiplying, and simplifying. It might take a bit of practice, but once you’ve got it down, you’ll wonder why you ever found it tricky!

Why Simplify After Multiplying?

Simplifying fractions after multiplying ensures your answer is in its simplest form. It’s like tidying up your workspace—it makes everything look cleaner and more organized. Plus, it’s just good math practice!

Dividing Mixed Numbers

Dividing mixed numbers is similar to multiplying, but with one key difference: you need to flip the second fraction (find its reciprocal) before multiplying. For example, to divide 3 1/2 by 1 1/4, first convert them to improper fractions (7/2 and 5/4). Then, flip the second fraction to get 4/5 and multiply: 7/2 × 4/5 = 28/10 = 2 8/10 = 2 4/5.

Dividing mixed numbers might seem complicated at first, but breaking it down step by step makes it manageable. And hey, practice makes perfect!

Tips for Dividing Mixed Numbers

Here are a few tips to keep in mind:

Always convert mixed numbers to improper fractions before dividing.
Don’t forget to flip the second fraction before multiplying.
Simplify your final answer if possible.

Real-Life Examples of Mixed Numbers

Mixed numbers aren’t just for math class—they’re everywhere in real life! Here are a few examples:

Baking: If a recipe calls for 2 1/2 cups of flour, you’re working with a mixed number.
Construction: Measuring materials often involves mixed numbers, like cutting a board to 6 3/8 inches.
Finance: Splitting bills or dividing profits can also involve mixed numbers, especially when dealing with partial amounts.

So next time you’re out and about, see if you can spot any mixed numbers in action. You’ll be surprised how often they come up!

How Mixed Numbers Make Life Easier

Without mixed numbers, we’d be stuck trying to explain quantities in awkward ways. Imagine saying “two wholes and one half” instead of just “two and a half.” Mixed numbers give us a concise, clear way to express parts and wholes, making communication and problem-solving a breeze.

Tips and Tricks for Working with Mixed Numbers

Here are a few tricks to help you master mixed numbers:

Always double-check your work, especially when converting between mixed numbers and improper fractions.
Use visual aids like number lines or fraction bars to help visualize what you’re working with.
Practice regularly—like any skill, working with mixed numbers gets easier with repetition.

And don’t forget: math is supposed to be fun! Embrace the challenge and enjoy the process of learning something new.

Overcoming Math Anxiety

If you find yourself getting nervous about math, you’re not alone. But here’s the thing: mixed numbers aren’t as scary as they seem. Break them down step by step, and you’ll start to see patterns and connections that make everything click. Trust me, you’ve got this!

Common Mistakes to Avoid

What Is A Mixed Number? Definition Examples, 48 OFF

Mixed Numbers worksheet Live Worksheets Worksheets Library

The Beginning Of Fatherhood