What Exactly Is The Definition Of A Mixed Number? A Deep Dive For Everyday Math Enthusiasts

Dr. Addie Funk 08 May 2025

Ever wondered what a mixed number really is and why it’s so important in math? If fractions have been giving you headaches, don’t sweat it. You’re not alone. Today, we’re diving deep into the world of mixed numbers, breaking them down in a way that even your math-phobic self can understand. Think of this as your ultimate cheat sheet to mastering mixed numbers, no stress involved.

Let’s face it, math can be tricky sometimes. But when it comes to understanding the definition of a mixed number, it’s all about perspective. Picture this: you’re baking a cake and the recipe calls for 2 and a half cups of flour. That “2 and a half” is actually a mixed number in disguise. Cool, right? Keep reading, because we’re about to make this concept stick in your brain like peanut butter on toast.

Whether you’re a student trying to ace your math test, a parent helping with homework, or just someone curious about numbers, this article has got you covered. We’ll explore everything from the basics to advanced applications of mixed numbers, sprinkling in some fun examples along the way. So, buckle up and let’s get started!

What is a Mixed Number?
Breaking It Down: Whole Numbers and Fractions
The History of Mixed Numbers
Real-Life Applications of Mixed Numbers
Converting Mixed Numbers to Improper Fractions
Adding Mixed Numbers Like a Pro
Subtracting Mixed Numbers: The Secret Sauce
Multiplying Mixed Numbers Without Breaking a Sweat
Dividing Mixed Numbers: The Final Frontier
Common Mistakes to Avoid
Tips for Learning Mixed Numbers

What is a Mixed Number?

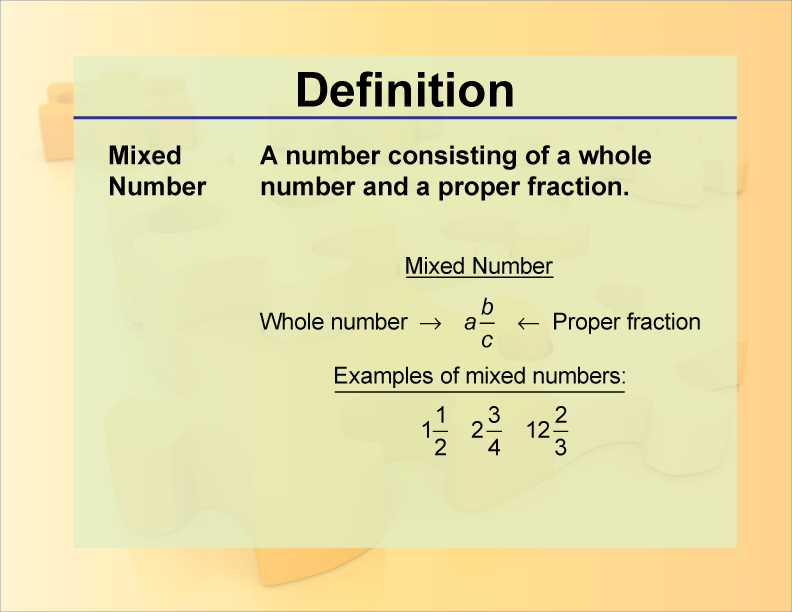
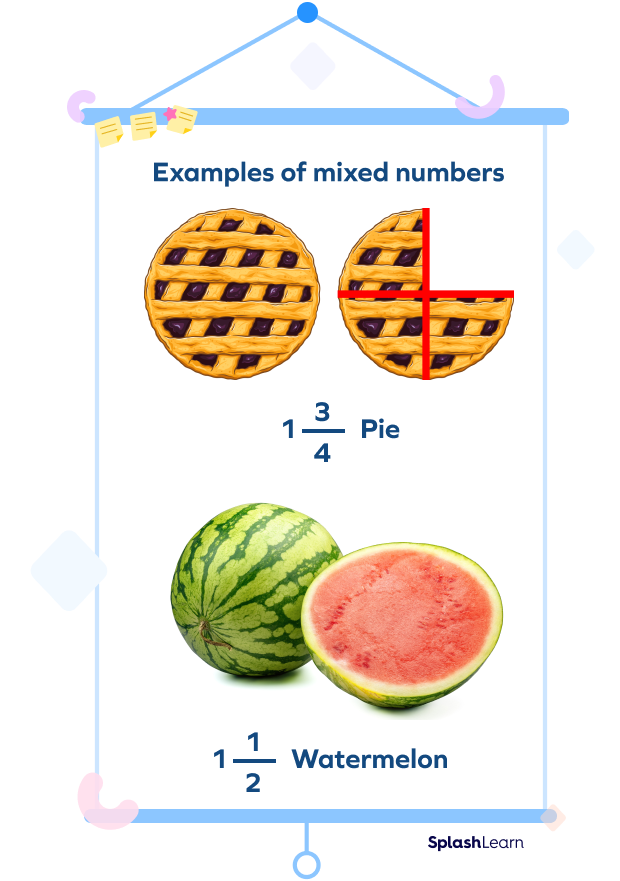
Alright, let’s get straight to the point. A mixed number is basically a combination of a whole number and a proper fraction. Think of it as a team effort between the two. For example, 3 ½ is a mixed number where 3 is the whole number and ½ is the fraction. Simple enough, right?

Now, here’s the kicker: mixed numbers are everywhere in real life. From measuring ingredients in recipes to splitting bills with friends, you’ll encounter them more often than you think. So, mastering this concept is like leveling up in the game of life.

Breaking It Down: Whole Numbers and Fractions

Understanding Whole Numbers

Whole numbers are like the OGs of the number world. They’re the ones you grew up with, like 1, 2, 3, and so on. No decimals, no fractions, just pure, wholesome numbers. When you combine them with fractions, you get mixed numbers.

Fractions: The Other Half of the Story

Fractions are where things get a little spicy. A fraction represents a part of a whole, like ½ or ¾. In a mixed number, the fraction is always proper, meaning the numerator (top number) is smaller than the denominator (bottom number). This keeps things balanced and manageable.

The History of Mixed Numbers

Believe it or not, mixed numbers have been around for centuries. Ancient civilizations like the Egyptians and Babylonians used fractions in their calculations, paving the way for what we know today. Over time, mathematicians refined the concept, making it an essential part of arithmetic.

Fast forward to modern times, and mixed numbers are still going strong. They’re taught in schools worldwide and used in countless real-world scenarios. So, the next time you use a mixed number, remember its rich history and the minds that made it possible.

Real-Life Applications of Mixed Numbers

Let’s talk about how mixed numbers show up in everyday life. Here are a few examples:

Cooking and Baking: Recipes often call for measurements like 1 ¾ cups of sugar.
Construction: Builders use mixed numbers to measure lengths, widths, and heights.
Shopping: Discounts and sales often involve fractions, like 25 ½% off.
Time: Clocks use mixed numbers to show time, such as 2:30 (or 2 ½ hours).

See? Mixed numbers aren’t just for math class. They’re a vital part of how we interact with the world.

Converting Mixed Numbers to Improper Fractions

Sometimes, it’s easier to work with improper fractions instead of mixed numbers. Here’s how you do it:

Multiply the whole number by the denominator.
Add the result to the numerator.
Keep the denominator the same.

For example, converting 2 ⅔ to an improper fraction:

2 × 3 = 6
6 + 2 = 8
Improper fraction: 8/3

Voilà! You’ve just turned a mixed number into an improper fraction. Practice this technique, and it’ll become second nature in no time.

Adding Mixed Numbers Like a Pro

Adding mixed numbers might sound intimidating, but it’s simpler than you think. Just follow these steps:

Add the whole numbers together.
Add the fractions together.
If the resulting fraction is improper, convert it back to a mixed number.

Example: Adding 1 ½ and 2 ⅓

Whole numbers: 1 + 2 = 3
Fractions: ½ + ⅓ = 5/6
Result: 3 ⅚

See? It’s not so bad. Keep practicing, and you’ll be adding mixed numbers like a pro in no time.

Subtracting Mixed Numbers: The Secret Sauce

Subtracting mixed numbers follows a similar process:

Subtract the whole numbers.
Subtract the fractions.
If the second fraction is larger, borrow from the whole number.

Example: Subtracting 3 ¾ from 5 ⅔

Whole numbers: 5 - 3 = 2
Fractions: ⅔ - ¾ = -⅙ (borrow 1 from 2, making it 1 ⅚)
Result: 1 ⅚

With a little practice, subtracting mixed numbers will feel as natural as breathing.

Multiplying Mixed Numbers Without Breaking a Sweat

Multiplying mixed numbers is all about converting them to improper fractions first:

Convert both mixed numbers to improper fractions.
Multiply the numerators together.
Multiply the denominators together.
Simplify the result if needed.

Example: Multiplying 2 ½ and 1 ⅓

Convert: 2 ½ = 5/2, 1 ⅓ = 4/3
Multiply: (5 × 4) / (2 × 3) = 20/6
Simplify: 20/6 = 3 ⅓

See? Multiplying mixed numbers is a piece of cake once you get the hang of it.

Dividing Mixed Numbers: The Final Frontier

Dividing mixed numbers is similar to multiplying, but with one extra step:

Convert both mixed numbers to improper fractions.
Flip the second fraction (find its reciprocal).
Multiply the two fractions together.
Simplify the result if needed.

Example: Dividing 3 ¾ by 1 ⅔

Convert: 3 ¾ = 15/4, 1 ⅔ = 5/3
Flip: 5/3 becomes 3/5
Multiply: (15 × 3) / (4 × 5) = 45/20
Simplify: 45/20 = 2 ⅕

Dividing mixed numbers might seem tricky at first, but with practice, it’ll become second nature.

Common Mistakes to Avoid

Here are a few pitfalls to watch out for when working with mixed numbers:

Forgetting to simplify fractions after calculations.
Messing up the borrowing process in subtraction.
Not converting mixed numbers to improper fractions before multiplying or dividing.

Stay vigilant, and you’ll avoid these common mistakes like a pro.

Tips for Learning Mixed Numbers

Mastering mixed numbers doesn’t have to be a chore. Here are some tips to make the process smoother:

Practice regularly with real-life examples.
Use visual aids like fraction bars or number lines.
Break down complex problems into smaller steps.
Seek help from teachers, tutors, or online resources if needed.

With a little effort and perseverance, you’ll become a mixed number wizard in no time.

Kesimpulan

And there you have it, folks! The definition of a mixed number isn’t as scary as it seems. By breaking it down into manageable parts and practicing regularly, you can master this concept and apply it to real-life situations with ease.

So, what’s next? Take action! Try solving a few problems on your own, share this article with a friend, or leave a comment below with your thoughts. Together, we can make math less intimidating and more approachable for everyone.

Remember, the world of numbers is full of possibilities. Embrace it, and who knows—you might just fall in love with math along the way. Cheers to mastering mixed numbers!

What Is A Mixed Number? Definition Examples, 48 OFF

The Beginning Of Fatherhood