What Does Mixed Number Mean? A Comprehensive Guide For Everyone

Robyn Rogahn 07 May 2025

So here's the deal, folks. You’ve probably come across the term "mixed number" at some point in your life, whether it was during math class or while trying to figure out how much pizza you actually ate last night. But what exactly does mixed number mean? Well, let me break it down for you in a way that’s super easy to understand, even if numbers aren’t exactly your thing. A mixed number is essentially a combination of a whole number and a fraction—think of it as a team effort between integers and fractions. It’s like when you have 2 full pizzas and half of another one. That right there? That’s a mixed number: 2 and 1/2.

Now, before we dive deeper into the world of mixed numbers, it's important to recognize why understanding them matters. Mixed numbers are everywhere! From cooking recipes to splitting bills with friends, they pop up in our daily lives more often than you might realize. By the end of this article, not only will you know what mixed numbers are, but you’ll also be able to work with them confidently. No more stressing over fractions or decimals—promise!

But hey, don’t just take my word for it. Let’s explore everything there is to know about mixed numbers, from their definition to practical applications, and even tips on how to simplify and convert them. So buckle up, because we’re about to embark on a mathematical journey that’s both fun and enlightening. Trust me, by the time you finish reading, you’ll wonder why you ever found math intimidating in the first place!

Understanding the Basics of Mixed Numbers

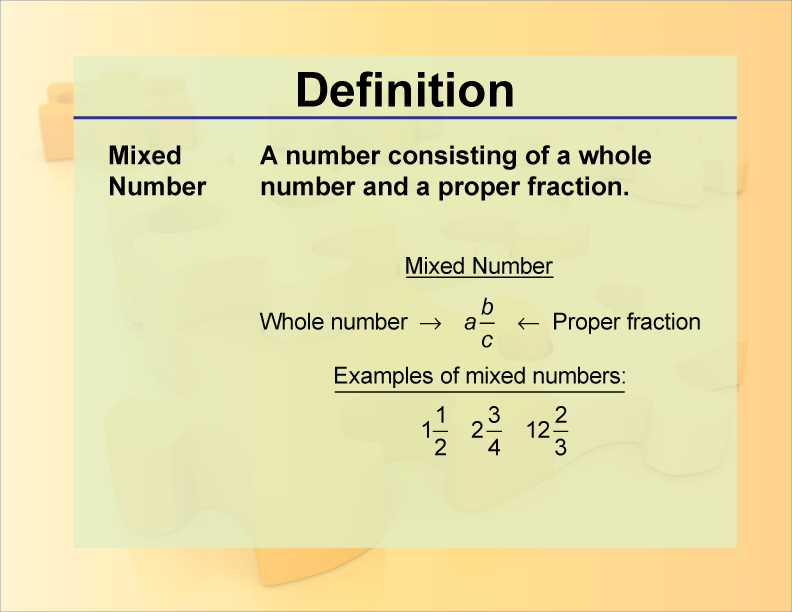
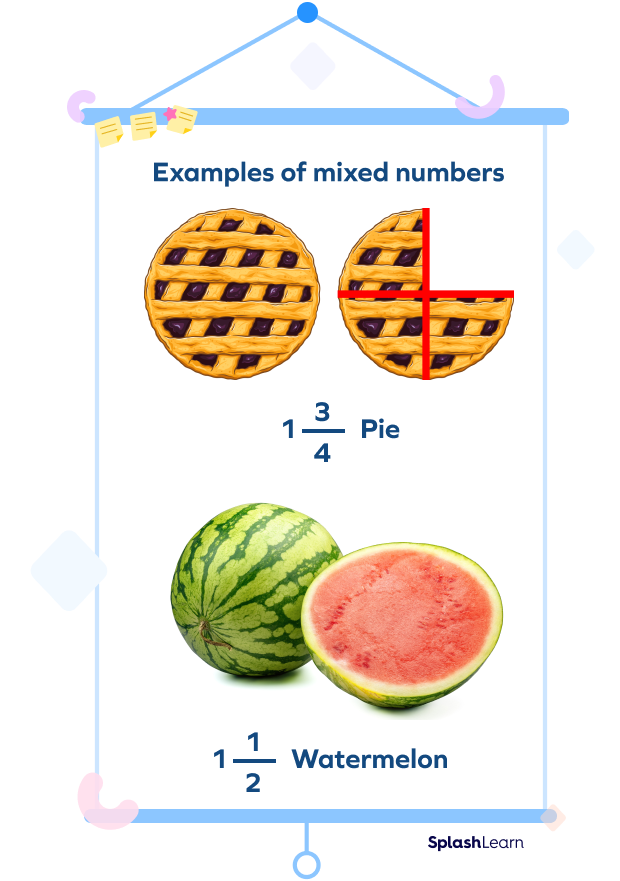
Alright, let’s start with the basics. A mixed number is basically a number that has two parts: a whole number and a fraction. It’s written like this: whole number + fraction. For example, 3 and 3/4 is a mixed number. The "3" represents the whole number, and the "3/4" represents the fractional part. It’s kind of like having three full apples and three-quarters of another one.

Here’s where things get interesting. Mixed numbers are used when you want to express a value that’s more than a whole number but less than the next whole number. Think of it as a middle ground between integers and fractions. For instance, if you’re measuring ingredients for a recipe and need 2 and 1/2 cups of flour, you wouldn’t just grab 2 cups or go all the way to 3 cups—you’d use a mixed number to get the exact amount.

Why Are Mixed Numbers Important?

Let’s face it, folks. Mixed numbers aren’t just random concepts invented by math teachers to torture students. They’re actually super useful in real life. Here’s why:

Cooking and Baking: Recipes often require measurements like 1 and 1/4 cups of sugar or 3 and 1/2 teaspoons of salt. Without mixed numbers, you’d either overdo it or fall short.
Construction and Carpentry: Builders and carpenters deal with measurements all the time, and mixed numbers help them get precise cuts and dimensions.
Finance and Budgeting: When splitting costs or calculating expenses, mixed numbers can make things clearer. For example, if you owe someone $15 and 50 cents, you could write it as 15 and 1/2 dollars.

How to Write Mixed Numbers Properly

Writing mixed numbers might seem straightforward, but there are a few rules to follow. First, always separate the whole number and the fraction with a space. For example, instead of writing "31/4," you write "3 1/4." This makes it easier to read and understand. Also, make sure the fraction part is simplified. So if you have 2 and 4/8, you’d simplify it to 2 and 1/2.

Common Mistakes to Avoid

Now, let’s talk about some common mistakes people make when writing mixed numbers:

Forgetting the Space: Writing "31/4" instead of "3 1/4" can confuse readers and lead to errors.
Not Simplifying Fractions: Leaving fractions in their unsimplified form, like 4/8 instead of 1/2, can make calculations more complicated.
Mixing Up Numerators and Denominators: Always double-check that the numerator (top number) is smaller than the denominator (bottom number) unless you’re dealing with improper fractions.

Converting Mixed Numbers to Improper Fractions

Sometimes, you’ll need to convert a mixed number into an improper fraction. Why? Because improper fractions are easier to work with in certain calculations, like addition and subtraction. Here’s how you do it:

Let’s say you have the mixed number 3 and 2/5. To convert it to an improper fraction:

Multiply the whole number (3) by the denominator (5): 3 × 5 = 15.
Add the numerator (2) to the result: 15 + 2 = 17.
Write the new numerator (17) over the original denominator (5): 17/5.

Voilà! You’ve converted 3 and 2/5 into 17/5.

Why Convert Mixed Numbers?

Converting mixed numbers to improper fractions is useful for several reasons:

Easier Calculations: Adding, subtracting, multiplying, and dividing fractions becomes simpler when they’re in improper form.
Standardization: Many math problems and equations require fractions to be in improper form for consistency.
Real-World Applications: In fields like engineering and physics, improper fractions are often preferred for precision and accuracy.

Adding and Subtracting Mixed Numbers

Adding and subtracting mixed numbers might sound tricky, but it’s actually pretty straightforward once you get the hang of it. Here’s how you do it:

Step-by-Step Guide for Addition

Let’s add 2 and 1/3 to 1 and 2/3:

Separate the whole numbers and fractions: (2 + 1) + (1/3 + 2/3).
Add the whole numbers: 2 + 1 = 3.
Add the fractions: 1/3 + 2/3 = 3/3 = 1.
Combine the results: 3 + 1 = 4.

So, 2 and 1/3 plus 1 and 2/3 equals 4.

Subtraction Made Easy

Now let’s subtract 1 and 1/4 from 3 and 3/4:

Separate the whole numbers and fractions: (3 - 1) + (3/4 - 1/4).
Subtract the whole numbers: 3 - 1 = 2.
Subtract the fractions: 3/4 - 1/4 = 2/4 = 1/2.
Combine the results: 2 + 1/2 = 2 and 1/2.

Easy peasy, right?

Real-Life Applications of Mixed Numbers

Mixed numbers aren’t just for math class—they’re everywhere in real life. Here are a few examples:

In the Kitchen

Cooking and baking often involve mixed numbers. For instance, if a recipe calls for 2 and 1/2 cups of flour, you’ll need to measure exactly that amount. Without mixed numbers, you’d either end up with too much or too little, and nobody wants a flat cake or overly dense bread.

DIY Projects

Whether you’re building furniture or fixing up your home, mixed numbers come in handy. Let’s say you’re cutting a piece of wood that needs to be 4 and 3/8 inches long. Having a clear understanding of mixed numbers ensures your cuts are precise and your projects turn out perfectly.

Common Challenges with Mixed Numbers

Even though mixed numbers are incredibly useful, they can sometimes be a bit tricky to work with. Here are a few common challenges and how to overcome them:

Dealing with Large Denominators

When fractions have large denominators, it can be tough to simplify them. For example, 7 and 19/24 might look intimidating, but you can simplify it by finding the greatest common divisor (GCD). In this case, the GCD of 19 and 24 is 1, so the fraction remains as is.

Handling Negative Mixed Numbers

Negative mixed numbers can be confusing at first, but they follow the same rules as positive ones. Just remember that the whole number and fraction are both negative. For instance, -3 and -1/4 is the same as -(3 + 1/4).

Advanced Topics: Multiplying and Dividing Mixed Numbers

Once you’ve mastered adding and subtracting mixed numbers, it’s time to move on to multiplication and division. These operations can be a bit more complex, but with practice, you’ll get the hang of them.

Multiplying Mixed Numbers

Let’s multiply 2 and 1/2 by 3 and 3/4:

Convert both mixed numbers to improper fractions: 2 and 1/2 = 5/2, 3 and 3/4 = 15/4.
Multiply the numerators: 5 × 15 = 75.
Multiply the denominators: 2 × 4 = 8.
Write the result as an improper fraction: 75/8.
Convert back to a mixed number if needed: 75 ÷ 8 = 9 with a remainder of 3, so the answer is 9 and 3/8.

Dividing Mixed Numbers

Dividing mixed numbers works similarly to multiplication. Let’s divide 3 and 1/2 by 1 and 1/4:

Convert both mixed numbers to improper fractions: 3 and 1/2 = 7/2, 1 and 1/4 = 5/4.
Invert the second fraction and multiply: (7/2) × (4/5) = 28/10.
Simplify the result: 28/10 = 2 and 8/10 = 2 and 4/5.

Tools and Resources for Learning Mixed Numbers

If you’re looking to improve your skills with mixed numbers, there are plenty of tools and resources available:

Online Calculators: Websites like Mathway and Symbolab offer calculators that can help you practice converting, adding, and multiplying mixed numbers.
Educational Videos: Platforms like YouTube have tons of tutorials on working with mixed numbers. Channels like Khan Academy and Math Antics are great places to start.
Math Apps: Apps like Photomath and Mathway allow you to scan math problems and get step-by-step solutions, making learning more interactive and engaging.

Tips for Mastering Mixed Numbers

Here are a few tips to help you become a pro at working with mixed numbers:

Practice Regularly: The more you practice, the more comfortable you’ll become with mixed numbers.
Use Visual Aids: Drawing diagrams or using fraction circles can make understanding mixed numbers much easier.
Break It Down: When faced with a complex problem, break it down into smaller steps. Focus on one part at a time to avoid feeling overwhelmed.

Conclusion

And there you have it, folks! A complete guide to understanding what mixed numbers mean and how to work with them. Mixed numbers might seem intimidating at first, but once you get the hang of them, they’re actually pretty straightforward. From cooking to construction, mixed numbers play a vital role in our daily lives, so mastering them is definitely worth the effort.

So, what’s next? Well, why not try practicing some mixed number problems on your own? Or better yet, share this article with a friend who could use a refresher. Remember, math doesn’t have to be scary—sometimes, all it takes is a little patience and practice

What Does it Mean to be Mixed? Mixed Millennial

What Is A Mixed Number? Definition Examples, 48 OFF

The Beginning Of Fatherhood