Mastering Mixed Numbers: A Comprehensive Guide To Boost Your Math Skills
Hey there, math enthusiasts! Let's dive into the fascinating world of mixed numbers. Whether you're a student struggling with fractions or someone brushing up on their math skills, this guide is here to help. Mixed numbers might sound intimidating, but trust me, they're easier than you think. Let's break it down step by step and make math fun again!
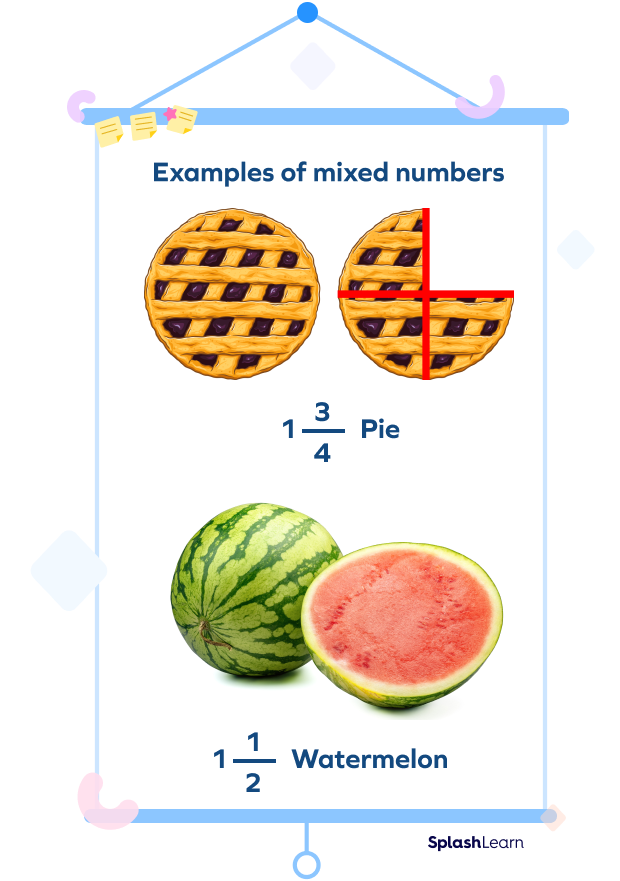
Mixed numbers are like the cool kids in the math world. They combine whole numbers and fractions into one awesome package. Think of them as the perfect blend of simplicity and complexity. Once you get the hang of it, working with mixed numbers will feel like second nature. So, let's get started and explore everything you need to know about mixed numbers.
This article is packed with practical tips, real-world examples, and step-by-step explanations. By the time you finish reading, you'll not only understand mixed numbers but also feel confident solving problems that involve them. So, grab your notebook, and let's conquer this math challenge together!
- Sza Porn Debunking The Myth And Understanding The Truth
- Unblocked Games Your Ultimate Guide To Fun And Entertainment
Table of Contents
- What Are Mixed Numbers?
- Converting Mixed Numbers
- Adding Mixed Numbers
- Subtracting Mixed Numbers
- Multiplying Mixed Numbers
- Dividing Mixed Numbers
- Real-World Applications of Mixed Numbers
- Common Mistakes to Avoid
- Tips for Learning Mixed Numbers
- Frequently Asked Questions
What Are Mixed Numbers?
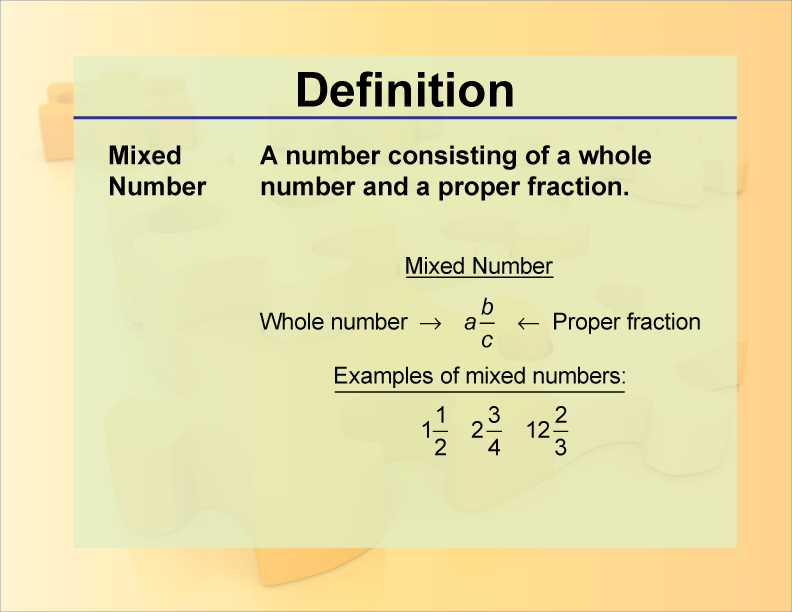
Alright, let's start with the basics. A mixed number is basically a combination of a whole number and a proper fraction. It's like having a pizza where you've eaten some slices but not the whole thing. For example, if you have 3 whole pizzas and 1/4 of another pizza, you'd write it as 3 1/4. Cool, right?
Here's the thing, mixed numbers are super useful in everyday life. Whether you're measuring ingredients for a recipe or calculating distances, mixed numbers come in handy. Understanding them is like unlocking a secret code in the math universe.
Structure of Mixed Numbers
Every mixed number has three parts: the whole number, the numerator, and the denominator. Let's break it down:
- Anjali Arora Viral Mms The Inside Story You Need To Know
- Paige Bueckers Nude Clearing The Misunderstandings And Focusing On Her Incredible Journey
- Whole Number: The number before the fraction. It tells you how many complete units you have.
- Numerator: The top number in the fraction. It shows how many parts you're dealing with.
- Denominator: The bottom number in the fraction. It indicates the total number of equal parts the whole is divided into.
For example, in 5 2/3, 5 is the whole number, 2 is the numerator, and 3 is the denominator. Easy peasy!
Converting Mixed Numbers
Now that you know what mixed numbers are, let's talk about converting them. Sometimes, it's easier to work with improper fractions instead of mixed numbers. Don't worry, it's not as complicated as it sounds.
Converting Mixed Numbers to Improper Fractions
To convert a mixed number to an improper fraction, follow these steps:
- Multiply the whole number by the denominator.
- Add the result to the numerator.
- Write the new number over the original denominator.
Let's do an example: Convert 4 1/5 to an improper fraction.
Step 1: Multiply 4 (whole number) by 5 (denominator) = 20.
Step 2: Add 20 to 1 (numerator) = 21.
Step 3: Write 21 over 5 = 21/5. Voilà!
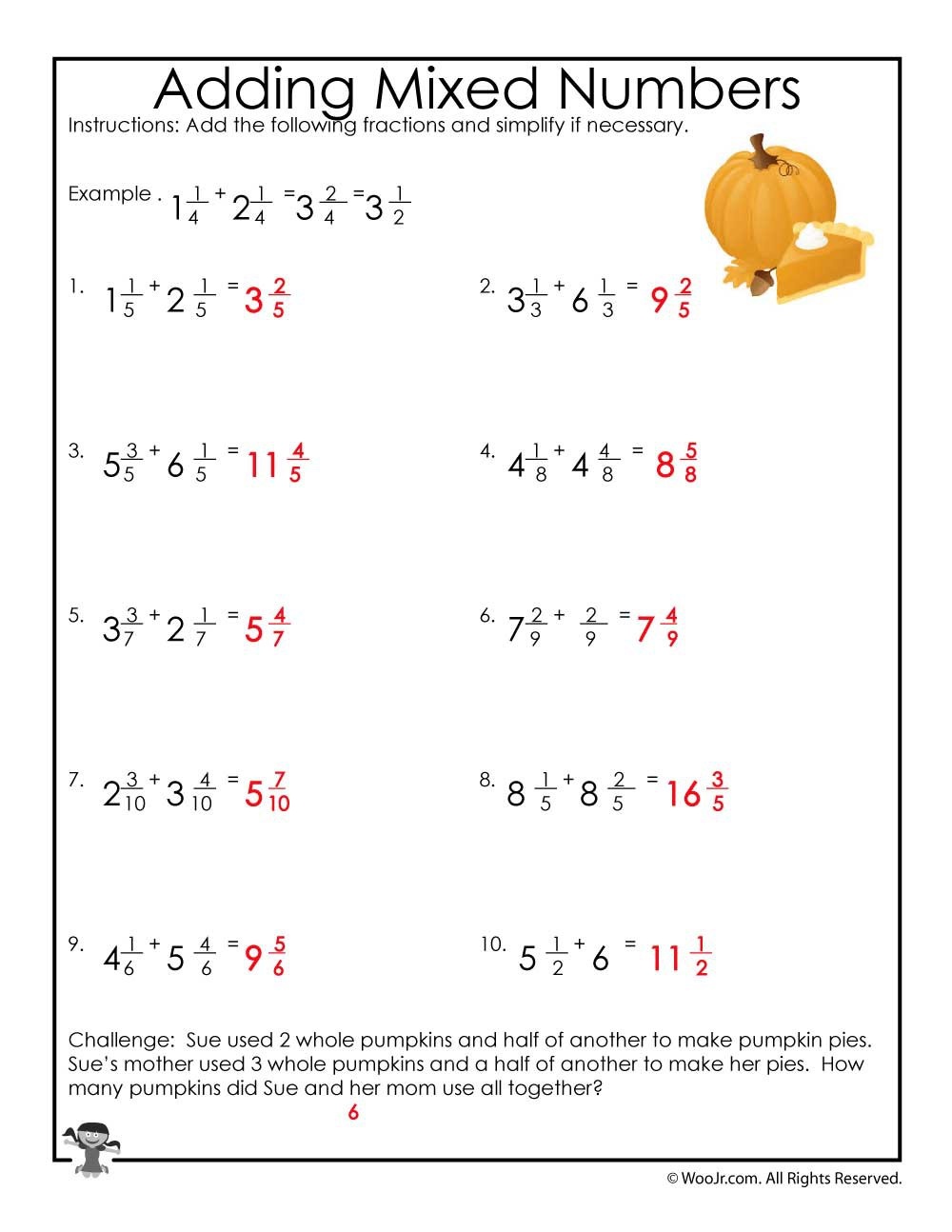
Adding Mixed Numbers
Adding mixed numbers can seem tricky at first, but with a little practice, you'll master it in no time. Here's how you do it:
Step-by-Step Guide
- Separate the whole numbers and fractions.
- Add the whole numbers together.
- Add the fractions together. If the denominators are different, find a common denominator first.
- Combine the results.
Let's try an example: Add 2 3/4 and 1 1/2.
Step 1: Separate the whole numbers and fractions: 2 + 1 and 3/4 + 1/2.
Step 2: Add the whole numbers: 2 + 1 = 3.
Step 3: Add the fractions. The denominators are different, so find a common denominator (4). Convert 1/2 to 2/4. Now, add 3/4 + 2/4 = 5/4.
Step 4: Combine the results: 3 + 5/4 = 3 5/4. Simplify if needed: 3 5/4 = 4 1/4. Done!
Subtracting Mixed Numbers
Subtracting mixed numbers follows a similar process to adding them. The key is to handle the fractions carefully. Here's how:
Step-by-Step Guide
- Separate the whole numbers and fractions.
- Subtract the whole numbers.
- Subtract the fractions. If the second fraction is larger, borrow from the whole number.
- Combine the results.
Let's do an example: Subtract 3 1/3 from 5 2/3.
Step 1: Separate the whole numbers and fractions: 5 - 3 and 2/3 - 1/3.
Step 2: Subtract the whole numbers: 5 - 3 = 2.
Step 3: Subtract the fractions: 2/3 - 1/3 = 1/3.
Step 4: Combine the results: 2 + 1/3 = 2 1/3. Simple as that!
Multiplying Mixed Numbers
Multiplying mixed numbers is a bit different. First, convert them to improper fractions, then multiply the numerators and denominators separately. Here's the process:
Step-by-Step Guide
- Convert the mixed numbers to improper fractions.
- Multiply the numerators.
- Multiply the denominators.
- Simplify the result if needed.
Let's multiply 2 1/2 by 3 1/4.
Step 1: Convert to improper fractions: 2 1/2 = 5/2 and 3 1/4 = 13/4.
Step 2: Multiply the numerators: 5 × 13 = 65.
Step 3: Multiply the denominators: 2 × 4 = 8.
Step 4: Write the result: 65/8. Simplify if needed: 65/8 = 8 1/8. Boom!
Dividing Mixed Numbers
Dividing mixed numbers is another skill that will make you a math rockstar. The trick is to convert them to improper fractions first. Here's how:
Step-by-Step Guide
- Convert the mixed numbers to improper fractions.
- Flip the second fraction (find its reciprocal).
- Multiply the first fraction by the reciprocal of the second fraction.
- Simplify the result if needed.
Let's divide 3 1/2 by 1 1/4.
Step 1: Convert to improper fractions: 3 1/2 = 7/2 and 1 1/4 = 5/4.
Step 2: Flip the second fraction: 5/4 becomes 4/5.
Step 3: Multiply: 7/2 × 4/5 = 28/10.
Step 4: Simplify: 28/10 = 2 4/5. Nice work!
Real-World Applications of Mixed Numbers
Mixed numbers aren't just for math class. They have plenty of real-world applications that make them super useful. Here are a few examples:
- Cooking and Baking: Recipes often require measurements like 2 1/2 cups of flour or 1 1/4 teaspoons of salt.
- Construction: Builders use mixed numbers when measuring lengths, such as 5 3/4 inches for cutting wood.
- Finance: Mixed numbers can represent interest rates or investment returns, like 4 1/2%.
By mastering mixed numbers, you'll be able to tackle these everyday situations with confidence.
Common Mistakes to Avoid
Even the best of us make mistakes when working with mixed numbers. Here are some common pitfalls to watch out for:
- Forgetting to convert mixed numbers to improper fractions before multiplying or dividing.
- Not finding a common denominator when adding or subtracting fractions with different denominators.
- Miscalculating when borrowing from the whole number during subtraction.
Stay sharp and double-check your work to avoid these errors. Practice makes perfect!
Tips for Learning Mixed Numbers
Learning mixed numbers doesn't have to be a chore. Here are some tips to make the process smoother:
- Use visual aids like fraction circles or number lines to help understand the concept.
- Practice regularly with worksheets or online quizzes to reinforce your skills.
- Break down problems into smaller steps to avoid feeling overwhelmed.
Remember, math is all about practice and persistence. Keep at it, and you'll see improvement in no time!
Frequently Asked Questions
What is the difference between a mixed number and an improper fraction?
A mixed number combines a whole number and a proper fraction, while an improper fraction has a numerator larger than or equal to the denominator. Think of it like this: mixed numbers are easier to visualize, while improper fractions are better for calculations.
Can mixed numbers be negative?
Absolutely! Just like whole numbers and fractions, mixed numbers can be positive or negative. For example, -3 1/2 is a perfectly valid mixed number.
Why are mixed numbers important?
Mixed numbers are important because they help us express quantities that are more than a whole but less than the next whole number. They're used in everyday life for measurements, cooking, and more.
How can I improve my skills with mixed numbers?
Practice, practice, practice! Use online resources, textbooks, or apps to work on problems involving mixed numbers. The more you practice, the more comfortable you'll become.
Conclusion
Well, there you have it—a comprehensive guide to mastering mixed numbers. From understanding what they are to applying them in real-world scenarios, you now have the tools to tackle any mixed number problem that comes your way. Remember, math is all about practice and persistence. Keep practicing, and you'll see improvement in no time.
So, what are you waiting for? Dive into the world of mixed numbers and unlock your math potential. Don't forget to share this article with your friends and leave a comment below if you have any questions. Happy calculating!
- Mila Ruby Onlyfans The Untold Story You Need To Know
- Amanda Cerny Onlyfans The Ultimate Guide To Her Success And Impact
Mixed Number Addition Worksheets Worksheet Hero
What Is A Mixed Number? Definition Examples, 48 OFF
What Is A Mixed Number? Definition Examples, 48 OFF